Numerical Methods for Guided Waves

Guided ultrasonic waves (GUWs) provide a highly efficient method for the non-destructive evaluation (NDE) and the structural health monitoring (SHM) of waveguides such as beam-like or plate-like structures. Compared to ultrasonic bulk waves, the use of GUWs provides: (a) longer inspection range, (b) larger versatility owing to dispersive and multimodal nature and (c) complete coverage of the waveguide cross-section. These advantages can be fully exploited only once the complexities of guided wave propagation are unveiled and managed for the given test structure.

These complexities include the existence of multiple modes that can propagate simultaneously, and the frequency-dependent velocities and attenuation (dispersive behavior). For example, the knowledge of the wave velocity is important for mode identification. Similarly, the knowledge of those mode–frequency combinations propagating with minimum attenuation losses helps maximizing the inspection coverage.

GUIGUW (Graphical User Interface for Guided Ultrasonic Waves) is an interactive program designed to calculate the dispersive properties of stress waves propagating in waveguides of any shape. Using GUIGUW, the user can easily identify and evaluate the possible acoustic waves existing in a wide range of structures. GUIGUW is developed in Matlab and compiled as a stand-alone software. The current version can be downloaded at www.guiguw.com and used for free.

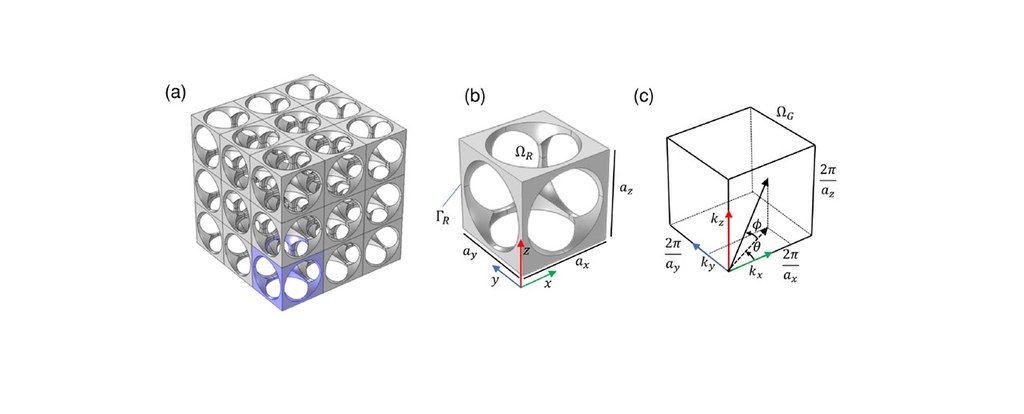
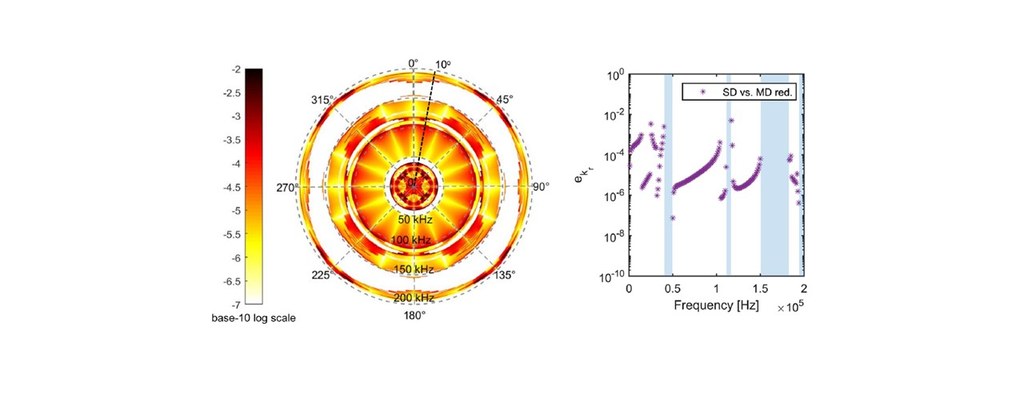
Periodic structures have the unique feature to inhibit the propagation of mechanical waves in certain frequency ranges, generally termed as band gaps. This appealing characteristic allows for the control of mechanical waves by designing passive mechanical filters as well as energy concentrators. This potential is hampered by the possibility of efficiently modeling and designing complex periodic materials by shaping their constitutive and geometrical properties. To this aim, fast and accurate computational methods, capable to handle proper rheological models, are needed. In this context our research contribution has been focused on the development of Reduced Order-Models for viscoelastic media to allow for a fast computation of the so-called complex-band structures in 1D, 2D and 3d periodic structures.

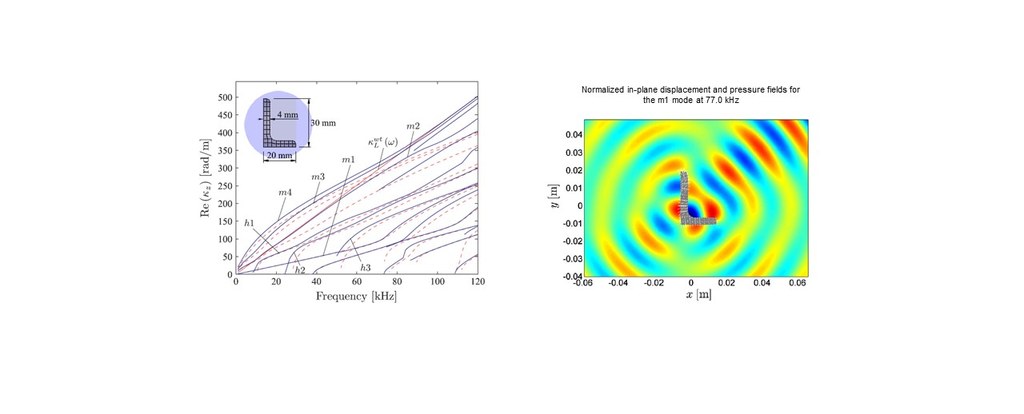
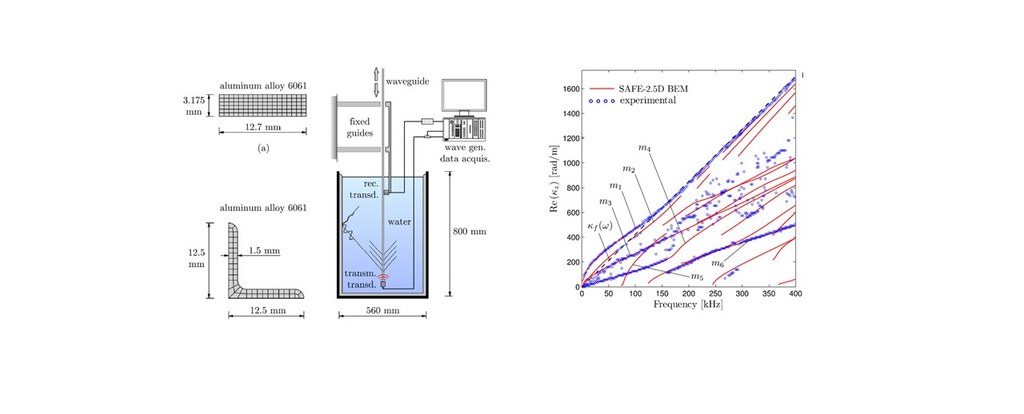
A challenging topic in wave propagation is the prediction of leaky waves in open waveguides (waveguides immersed in fluids or solids) and cavities. This problem is generally tackled by the research community exploiting perfectly matching layers (PML) approaches by accepting their limitations in the approximation of the problems. Our research contribution in this line has been in the development of coupled SAFE-BEM algorithms, where the BEM grid at the contour of the waveguide rigorously models the surrounding media. The efficiency and accuracy of SAFE-BEM algorithms are still unmatched as they tackle the problem of leaky guided waves in open waveguides and provides benchmark solutions for the research community. The developed formulations are in use in several research projects by several research groups.